The chi-square test of independence is used to test whether two populations or variables are related or independent to each other with
respect to some characteristic.

1. Know when to use the chi-square test for independence.

The chi-square test of independence is used to test when two categories (each with many cells or groups) are related or not related 
(independent).

Sometimes individual items are classified into categories in terms of two different criteria. For example, in studying the wear of tires, tires
may be classified into two categories: (1) front and rear as well as (2) left and right. A detail examination of both classification yields a 
matrix or table of possible combinations of the classifications:

contingency table
 

Category 1	Category 2		Row Total
	Front (F)	Rear(B)
Left (L)	fLF	fLB	fL (Left Total)
Right (R)	fRF	fRB	fR (Right Total)
Column Total	fF (Front Total)	fB (Rear Total)	n (Total of All Categorized Cells)

The table above where categorical cells are combined to show their rows and column totals is called a contingency table.

Example 2 (from Textbook). For two categries: (1) Political candidates, A and B and (2) Voter Types, Male or Female a reporter 
may summerize a survey of perference for either candidates as follows (contingency table):
 

Voters	Candidates A	Candidates B	Total Voter Types
Female	Female Votes for A	Female Votes for B	Female Votes
Male	Male Votes for A	Male Votes for B	Male Votes
Total: Votes per Candidates	Votes for A	Votes for B	All Votes

2. Know how to setup a Contingency Tables for the chi-square tests when studing independence (or homegeneity ) of 
categorical data.

A contingency table organizes the results of a study involving two categoriies (with many classifications within each category) by 
showing the frequency for each subtotals (groups, classes or cells) within each category.

A contingency table (Observed frequencies) is constructed as followed:
 

Criterion A	Criterion B				Row Total
	1	2	.....	k
1	f11	f12	.....	f1k	Row1
2	f21	f22	.....	f2k.	Row2
.	.	.	......	.	.
.	.	.	......	.	.
m	fm1	fm2	.....	fmk	Rowm
Colum Total	Column1	Column2	.....	Columnk	n (Grand Total)

Where:

m=	The number of classes of grouping in category A (Row Classification)
k=	The number of classifications of category B (Column Classification)
Rowi =	Total of row frequencies (observed)
Columnj =	Total of column frequencies (observed)
fij	Number of items or results that belong to both the i-th and j-th categories For example, for f12 i=1 and j=2 so is the frequency observed for both criteria when Criterion A is 1 and Criterion B is 2.
n=	Grand total (total number of observations)

3. Know how to compute the expected freguencies for chi-square test of independent charateristics.

Each cell in the contingency table have some theoretical or expected frequency and unless otherwise known (explicitly) it is determined 
from the information given in the row, column and grand totals.

The expected frequency, E for each cell is determined by taking the product of the corresponding row and column totals divided by the 
grand total.

4. Know the relationship between the observed and expected frequencies and the chi-square test of independence.

The chi-sqaure test for independence evaluates the closeness of the observed frequencies (O) f_ij to the expected frequencies (E), E_ij, 
good agreement between these two information favors the null hypothesis and large departure of the observed frequencies from the expected 
frequencies favors the alternate hypothesis (larger values of ). 

Remember the chi-square statistics is 

5. Know the multiplication rule for independence characteristics and its role in chi-square analysis.

The cell frequencies found in the contingency table when divided by the grand total yield the proportions, p of observations or results out 
of the total number of observations. This proportions is also a probability score of cells posibilities out of total possible outcome or score.

If p(m) is the proportion of row_m (frequency of row_m divided by the grand total and p(k) is the column proportion of column_k (probability, p_k) 
then:

The multiplication rule for independent characteristics is p(m and k) = p(m) x p(k).

Where p(m and k) or p(mk) = is the probability or frequency of both m and k occuring (assumes that both m and k are indepedent events 
or charateristics)

Example (Observed Proportion - from table below) 

( Expected Proportion - from table below) 

The chi-square test of independence attempts to compare the expected proportions using the multiplication rule of independent characteristics 
with the actual or observed proportions in each cell of the contingency table.

If the multiplication rule does not hold for each cell, the two charateristics are not independent, they are related.

That is, the sum of square of the Observed frequencies minus the expected is small.

I is small.

6. Know how to compute and evaluate the chi-square test of independence statistics.

Problem: A researcher wants to study how 7 methods of preparations affects students getting over 80% on a aptitude test. The researcher 
would also like to know how elapsed time after preparation affects student's performance on the test (> 80%) after 1, 2 and 3 months of final 
preparation and if these two criteria are related or independent.

The following table is the observed results of the study: contingency table.

Use chi-square test for independent charateristics to evaluate this data.
 

Number of Months (M) after Prep.	Methods of Prep. (preperation)							Row Totals
	1	2	3	4	5	6	7
1. After 1 M	97	8	18	8	23	21	5	180
2. After 2 M	120	15	12	13	21	17	15	213
3. After 3 M	82	4	0	12	38	25	19	180
Column Totals	299	27	30	33	82	63	39	573

Procedure for calculating chi-square test of independence:

Step 1. Make a problems statement: (becomes the hypothesis statement, H_o ).

(1) Are the Methods of preperation and number of months after preperation related in terms of students achievement scores (> 80% on test)?

(2) Are the two criteria (methods of Preperation and number of months test is taken after preperation) independent or related to with respect 
to students performance scores (>80% on test)?

Note: that the formulation of a problems statement or the question that the research would like to answer statistically may take on many forms 
but similar expectations. For both problems statements above (1) and (2) are inversely related for when on is true the other is false and when on is 
false the other is true. Either statement answers the larger question of indepedence: Yes of No?

Hypothesis:

So H_o (null hypothesis): Methods of Prep and Months after Prep are independent. (not related or interacts with respect to students scores).

H_a: H_o is not true. (alternate hypothesis): Methods of Prep and Months after Prep are related.

Step 2. Choose , the significance level of the test.

If you want the be 95 % certain that the test is true, then  = 0.05 =(100-95)/100

The df = (r-1)(c-1), or (m-1)(k-1) = (3-1)(7-1)=12

So df = 12

Step 3. Look up  from chi-square table:
 

df
12	3.07	3.57	4.4	5.23	6.3	18.55	21.03	23.34	26.22	28.3

For d.f. = 12, 

Step 4. (4) Determine or compute , The Expected Frequencies:

The following table is the Expected Frequencies, E of each cells in the study: 
 

Number of Months (M) after Prep.	Methods of Prep. (preperation)							Row Freq. Total
	1	2	3	4	5	6	7
1	93.9267	8.4817	9.4241	10.3665	25.7592	19.7906	12.2513	180
2	111.1466	10.0366	11.1518	12.267	30.4817	23.4188	14.4974	213
3	93.9267	8.4817	9.4241	10.3665	25.7592	19.7906	12.2513	180
Column Freq. Totals	299	27	30	33	82	63	39	573

Step 5. Compute 

Table of Expected Values (click for larger image)
 

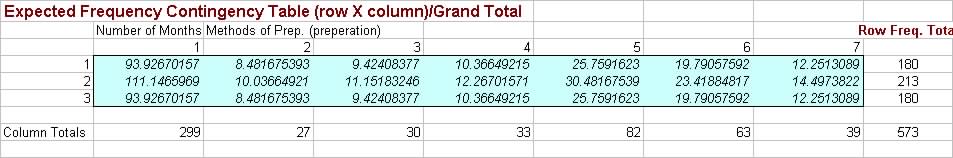(click for larger image)
 

= 45.5974 from computational table above.

Step 6 Perform test chi-square test: , 

Since , i.e. 45.60 > 21.03, Then we assume that the Null Hypothesis is not true (the types of preparations and number of weeks 
after preperation are related each other).

Make Conclusion or inference:

There seems to be some relationship between types of students' preparation and number of weeks after preparation that tests are taken.

So H_a (alternate hypothesis) is favored by this test.

Workshop Problem: (Test of Independence)

Formulate an hypothesis about the relationship between Political view and Opinion of Nuclear Power from the following survey of 100 students. 
Use a chi-square test for independence showing contingency table to evaluate your hypothesis and make inference about the results of this study.
 

Opinion	Political Views
	Democrat	Republican	Independent
Approve	10	15	20
Disapprove	9	2	16
Undecided	8	2	18

(a) What is your hypothesis?

(b) What is the value of the chi-square statistics?

(c) What conclusion did you make?

(d) What inference can you make?

Use use contingency table to help evaluate.