The chi-square test for homogeneity is a test made to determine whether several populations are similar or equal or homogeneous in 
some characteristics.

1. Know what is meant by the test for homegeneity.

The test for Homogeneity is evalauting the equality of several populations of categorical data. The test asked whether 3 or more
populations are equal with respect to some characteritics.

The homogeneity chi-square test statistics is computed exactly the same as the test for independence using contingency table as 
when determining the independence of charateristics chi-square statistics.

The only difference between the test for independence and the homogeneity test is the stating of the null hypothesis:

Homogeneity tests a null hypothesis asserting that various populations are homogeneous or equal with respect to some charateristics 
of interest against an alternate hypothesis claiming that they are not.

If  is populations i-th (categorized) then  (the null hypothesis)

(note other statistics could be used to compare pairwair combinations of all possible pairs; however if there is a mistake in judging the 
goodness of one or more of the pairs, then the error introduced will be too large to effectively make as good a claim as the chi-square
homegeneity test would.)

2. Know how to compute the chi-square homegeniety test statistics.

Problem: An ads agency wishes to determine if there are any differences with respect to reader recall among 3 kinds magazine ads. 
One ad is humorous, the second is quite technical and the third is a pictorial comparision of competing brands. Appropriate random
sampling, response validations are taken and conducted to determine how well partiscipants remembered each ads on a national / regional 
level. Those partiscipants selecting the correct ad are labeled as remembered and those unable to select the correct ads are labeled 
nonremembered.
 

	Type of Ads
Reader Recall	1. Humorous	2. Technical	3. Comparison
1. Rememebered	25	10	7
2. Not Rememebered	73	93	108

Step 1. Make a problems statement: (becomes the hypothesis statement, H_o ).

The main question to answer is: are there any differences in recalling property (mnemonic) of the three kinds of advertisements? 
This may be expressed as proportions of readers who either remembered or not rememberd the ads.

Hypothesis:

If  represent the proportion rememebering the i-th ad (i=1 for Humorous, i=2 for Technical and i=3 for Comparision) then 
the null hypothesis is:

The ads make no difference in (helping) reader recall.

H_a: H_o is not true. (alternate hypothesis): There is a difference between the ads in terms of reader recall.

Step 2. Choose , the significance level of the test.

If you want the be 95 % certain that the test is true, then  = 0.05 =(100-95)/100

The df = (r-1)(c-1), or (m-1)(k-1) = (3-1)(2-1)=2

So df = 2

Step 3. Look up  from chi-square table:
 

df
2	0.01	0.02	0.05	0.1	0.21	4.61	5.99	7.38	9.21	10.6

For d.f. = 12, 

Step 4. (4) Determine or compute , The Expected Frequencies:

The following table is the Expected Frequencies, E of each cells in the study:
 

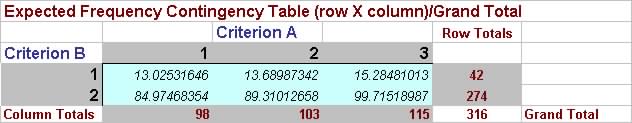Step 5. Compute 
 

= 19.02221 from computational table above.

Step 6 Perform test chi-square test: , 

Since , i.e. 19.0221 > 5.99, Then we assume that the null hypothesis of equaly proportions must be rejected 

Make Conclusion or inference:

We conclude that the three ads are not equally easy to remembered.

So H_a (alternate hypothesis) is favored by this test.

3. Know how to calculate the contingency coefficient, C.

The contingency coefficient, C is a statistics that measures the strength of the relationship between variables or categories in a 
contengency table of the chi-square statistics when the test suggest that variables or categories are related.

It is interpretated like the correlation coefficient.

, where  is the chi-square statistics obtained for the contingency table and n is the grand total of all frequencies.

This statistics is rarely used by practitioners.

Workshop Problem (test for Homogeneity)

A dietician wants to know if the time of day influences the tendency to consume coffee. The following data represents the beverage
purchases for a random sample of cafeteria customers.
 

	Early a.m.	Late a.m.	Early p.m.	Late p.m.
Number for coffee	3	5	8	11
Number for other	52	48	51	47

The null huypothesis is that the proportions of coffee purchases throughout the day are identical. Using alpha of 0.05, what conclusion 
should the dietician reach?